Runoff Routing Methods

6. Chapter 6 of 6. RUNOFF ROUTING METHODS

6.1 General

For larger catchments runoff routing methods are becoming widely adopted for hydrologic investigation for flood estimation. These methods involves determining the rainfall-excess and routing it through a model of the catchment storage by flood routing procedure. Computer models are invariably used due to the level of detail necessary in modelling the distributed nature of the catchment storage. Catchment storage includes the temporary storage of runoff in the stream channels and floodplains and on ground surface as well as longer term storage in reservoir and retarding basins. Most runoff routing models involves the modelling of the two dominant hydrologic processes of catchment losses (to determine the rainfall excess hyetograph) and runoff routing.

Runoff routing models allow the user to sub-divide the catchment into a number of sub-catchments to model the runoff generation and flow routing. This allows for the accounting of areal distribution of rainfall, land use, catchment and stream characteristics as well as future modifications to these parameters. Runoff routing models were developed to overcome some of the inherent problems associated with the Unit-hydrograph method such as areal lumping of catchment and rainfall characteristics as well as the utilisation of system linear theory. Research studies have found catchment flood response to be non-linear and runoff routing methods allow (to some degree) for the modelling the nonlinearity in catchment response. Studies have shown that there may be a need for further improvements in current runoff routing models to account for non-linearity in rivers with extensive floodplains.

A number of runoff routing models are currently available for urban and rural flood estimation and include ILSAX, RORB, RAFTS and WBNM. These models and others are discussed in some detail and various other runoff routing methods are described in detail in Book V of ARR (2001).

Regionally based Rainfall losses for Western Australia are provided in ARR Book Two, Table 3.2 and were derived for Runoff Routing (RORB) modelling from gauged catchments to produce a flow of the same recurrence interval to the rainfall. These losses should be identified as they form the best guide to the required losses to best model a catchment. It must be noted that the warning provided in ARR identifies that caution is required for the use of this data outside of the areas of determination. Losses in the catchment should be discreetly estimated taking into account not only infiltration and evaporation but also shape.

6.2 Basis of Runoff Routing

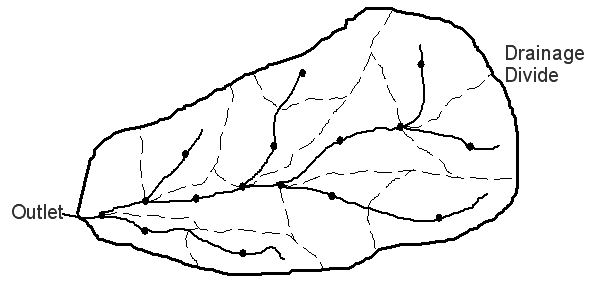
The establishment of a runoff routing model of a catchment commences with the sub-division of the catchment into sub-areas according to the stream network, catchment topography and land use, rainfall variability and location of interest. A node is assigned to the centroid of each sub-catchment and at the confluence of stream. Figure 6.1 illustrates a typical catchment sub-division for a runoff routing model.

A design or historic rainfall pattern is assigned to each sub-catchment node and the resulting rainfall excess determined. In a design situation, often one common design storm pattern would be assigned to sub-catchments. In the case of reproducing a historical storm event the number of different storm patterns applied to the catchment would be dependent on the data available.

While having the same storm pattern, most runoff routing models also allow different total storm rainfall to be assigned to each sub-catchment. It is quite common for sub-catchments to be assigned different storm depth while having the same storm temporal pattern to represent topographic effects on areal distribution of rainfall.

6.3 Loss Modelling

A rainfall excess hyetograph from each sub-catchment is calculated by applying the catchment loss model to each sub-catchment. The loss models in all runoff routing models consist of a combination of pervious area loss modelling and impervious area loss modelling. Common types of loss modelling for pervious areas include the initial loss/continuing loss model, the initial loss/runoff coefficient model, the Green-Ampt loss model and the Horton Infiltration Loss model. The most common loss model for impervious areas is simply a runoff coefficient model (usually with a runoff coefficient of 0.9). The user defines the land use in each sub-catchment by adjusting the proportion of pervious and impervious area.

The rainfall excess from each sub-catchment is then routed from the centroid of that sub-catchment, along the main stream, to the next downstream node where the runoff hydrograph is combined with (i) runoff hydrographs from other tributaries and/or (ii) rainfall excess hyetograph from the sub-catchment of the downstream node reach. The combined runoff hydrograph is then routed downstream to the next node and so on.

6.4 Flow Routing

Catchment storage has the effect of modifying the runoff hydrograph such that the peak flow is attenuated and translated in time as illustrated in Figure 6.2. This effect can be simulated by flood routing technique in which the input (i.e. rainfall excess hyetograph or the runoff hydrograph from an upstream node) is routed through a series of catchment storages.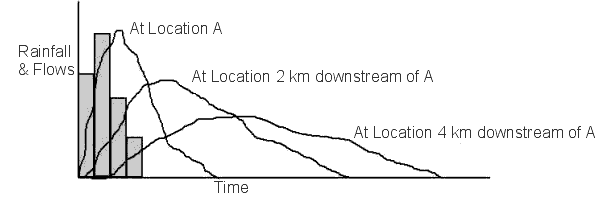

Figure 6.2 Illustration of Storage Effects on Flood Hydrographs

Most runoff routing model utilises a simple storage routing algorithm based on the continuity equation and a storage equation of the general form S = kQ^m. The storage equation is non-linear and this form of the storage equation is justifiable by both theoretical and empirical means. Theoretical derivation using a simple flow formula such as the Manning's Equation has shown that the parameter k represents the effect of such river characteristics as the hydraulic roughness, bed slope, length and cross section geometry.

The value of the exponent m, which affects the degree of non-linearity of the catchment flood response, has been shown to be related to the shape of the river channel with typical values of m being 0.6 for wide rectangular channel (i.e. under fully developed floodplain flow conditions perhaps) and 0.75 for triangular or trapezoidal sections. Like any models (no matter how simplified), the parameters of the model needs to be calibrated. The parameters k and m are calibrated from observed flood events and thus the reliability of the method reduces when the availability and the quality of calibration data are limited. Figure 6.3 below depicts the results of a calibration run of the RORB model.

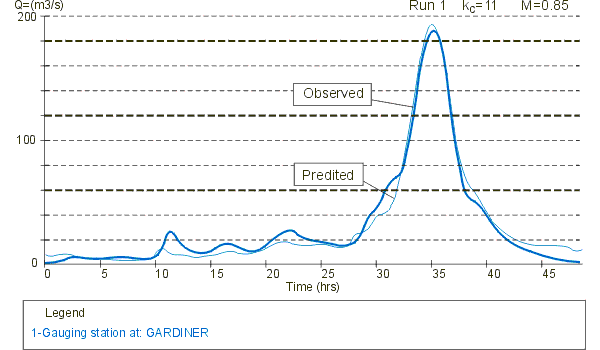
Figure 6.3 Typical Output from a RORB Model

6.5 Runoff Routing Parameters for Ungauged Catchment

For an ungauged catchment, the use of regional data is often adopted and the results are usually expected to have a higher uncertainty. The recognition that catchment flood response is non-linear in nature in runoff routing has nevertheless improved the reliability of extreme flood prediction, especially when recorded events for model calibration have high probabilities of exceedence. The traditional approach of the Unit-hydrograph method has been shown to have the tendency to under-estimate extreme floods in such circumstance. As mentioned earlier, the degree of non-linearity and the appropriate form of the storage equation (other than the power function commonly assumed in the industry) is still a subject of intense research.

The list of formulae for deriving regional values of the RORB catchment parameter kc for Western Australian catchments are described in Book V Volume 1, p 64, ARR (2001).

References

Australian Rainfall And Runoff; A Guide to Flood Estimation, Volume 1, 2001.

Australian Rainfall And Runoff; A Guide to Flood Estimation,Volume 2, 1987.

Laurenson, E. M. and Mein, R. G. RORB Version 3 Runoff routing program programmer manual,1986.